Topic 7: How do dislocations move in a material?

Overview

The movement of dislocations produces plastic strain in a material, and dislocations move in response to stresses; the Peach-Koehler equation $\mathbf{dF}=(\underset{―}{\sigma }\mathbf{b})\times \mathbf{dt}$ gives the force dF per length dt on a dislocation based on the stress $\underset{―}{\sigma }$. The plastic strain rate $\dot{\gamma }$ is derived from a density of dislocations ${\rho }_{\perp }$ with Burgers vector $b$ and velocity $v_{\perp }$ via the Orowan equation as $\dot{\gamma }=b{\rho }_{\perp }{v}_{\perp }$. Thus, plastic strain response of a material to stress is “merely” a matter of determining how dislocations move in a material. At the simplest level, dislocations have a minimum stress (the “Peierls stress”) required to initiate movement. Beyond that, it can be difficult to quantitatively measure the relationship between stress (or force) and dislocation velocity in a material.

For materials with very low Peierls stresses (such as most face-centered cubic metals), the dislocation mobility is primarily dominated by phonon drag. However, for body-centered cubic metals or other materials with high Peierls stresses, other defects are required for dislocations to move: pairs of kinks are nucleated, and move along the dislocation. Other processes, like cross-slip of screw dislocations, also require the formation and movement of kinks. Furthermore, climb motion of edge dislocations involve the elimination of vacancies at jogs. Thus, for many situations, dislocation motion requires other “defects.”

Reading

For this topic, you’ll want to review a few computational results on dislocation mobility and kinks in body-centered cubic materials, along with a paper on solid solution effects to have a sense of what is now feasible for computational studies of kinks.

• S. Queyreau, J. Marian, M. R. Gilbert, and B. D. Wirth, “Edge dislocation mobilities in bcc Fe obtained by molecular dynamics.” Phys. Rev. B 84, 064106 (2011): doi:10.1103/PhysRevB.84.064106
• T. D. Swinburne, S. L. Dudarev, S. P. Fitzgerald, M. R. Gilbert, and A. P. Sutton, “Theory and simulation of the diffusion of kinks on dislocations in bcc metals.” Phys. Rev. B 87, 064108 (2013): doi:10.1103/PhysRevB.87.064108
• Y.-J. Hua, M. R. Fellinger, B. G. Butler, Y. Wang, K. A. Darling, L. J. Kecskes, D. R. Trinkle, Z.-K. Liu, “Solute-induced solid-solution softening and hardening in bcc tungsten.” Acta Mater. 141, 304-316 (2017): doi:10.1016/j.actamat.2017.09.019

Team assignment

Thermally-activated slip (the primary deformation mode for $T\lesssim 0.15T_{\text{melt}}$) in BCC metals occurs via the nucleation of kink pairs, followed by the motion of the kinks along $\frac{a}{2}⟨111⟩$ screw dislocations. This physical model has been the basis for discrete dislocation dynamics computation of BCC metals through dislocation mobility, as well as our understanding of solute interactions with dislocations in BCC metals, such as solid-solution softening. An interesting new class of BCC alloy are multi-principal element alloys; TiZrNbHfTa is one such example. Your team has decided to try to build a numerical model of yield strength as a function of temperature and strain rate for this material.

1. What computational method(s) would you plan to use for this problem, and what might you find?
2. What experiment(s) would you suggest to provide either validation or additional information?

Prelecture questions

1. Jogs are formed when one dislocation cuts through another. Can kinks be formed this way as well? Either provide an example of how this could happen, or explain why it cannot.
2. Explain the role of jogs in dislocation climb. Can you write down an expression for dislocation climb velocity in terms of jog density, excess (above equilibrium) vacancy density, and any other quantities you believe to be relevant?
3. Derive an estimate of kink energy and width using the Peierls-Nabarro model combined with line energy. The most straightforward way is to consider a dislocation line that is straight, except for a kink segment; treat this kink as a straight line 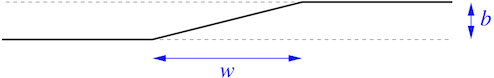where the straight segments sit in Peierls “valleys” of zero energy separated by a distance $b$, and the kink of width $w$ crosses between the two valleys. If the dislocation position in the valley is given by $u(x)$, then the Peierls energy (slip energy as the dislocation moves from one valley to another) is $${\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}\frac{W_{\text{P}}}{2}\{1-\cos \left(\frac{2\pi u(x)}{b}\right)\}dx.$$ for a Peierls barrier $W_{\text{P}}=b^2{\tau }_{\text{P}}/\pi$ and Peierls stress ${\tau }_{\text{P}}$. The other contribution to the energy is the line energy, which is given as an energy per length of $W_0\approx \frac{1}{2}G{b}^2$.

Suggested background

These may help you think about the papers and questions raised; you may want to look beyond these, too.

• Course webnotes:
  • 5.3.1 Movement, kinks, jogs, generation
  • 5.3.3 Climb of dislocations
• D. Hull and D. J. Bacon, Introduction to Dislocations. Fifth ed. (Elsevier, 2011): doi:10.1016/B978-0-08-096672-4.00003-7 Chapter 3.
• D. Hull and D. J. Bacon, Introduction to Dislocations. Fifth ed. (Elsevier, 2011): doi:10.1016/B978-0-08-096672-4.00007-4 Chapter 7.
• Slides (on Google Drive):
  • FCC Dislocations
  • Other Crystal Dislocations
  • Kinks and Jogs

Discussion: Nov. 12-14, 2024